Discrete distributions
Introduction to Statistics in Python

Maggie Matsui
Content Developer, DataCamp
Rolling the dice
Rolling the dice
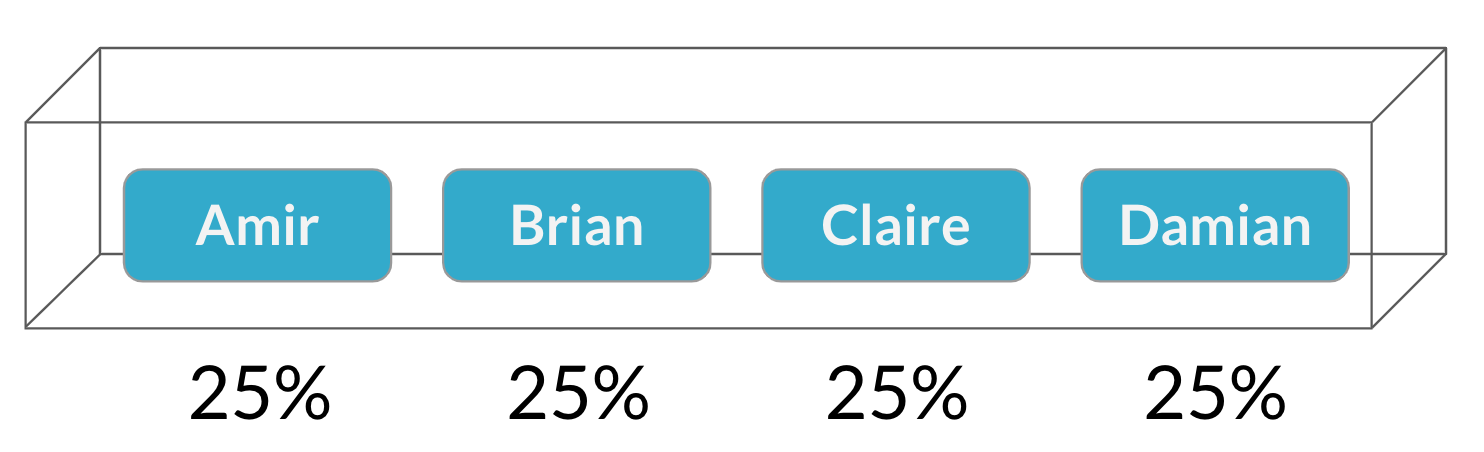
Choosing salespeople
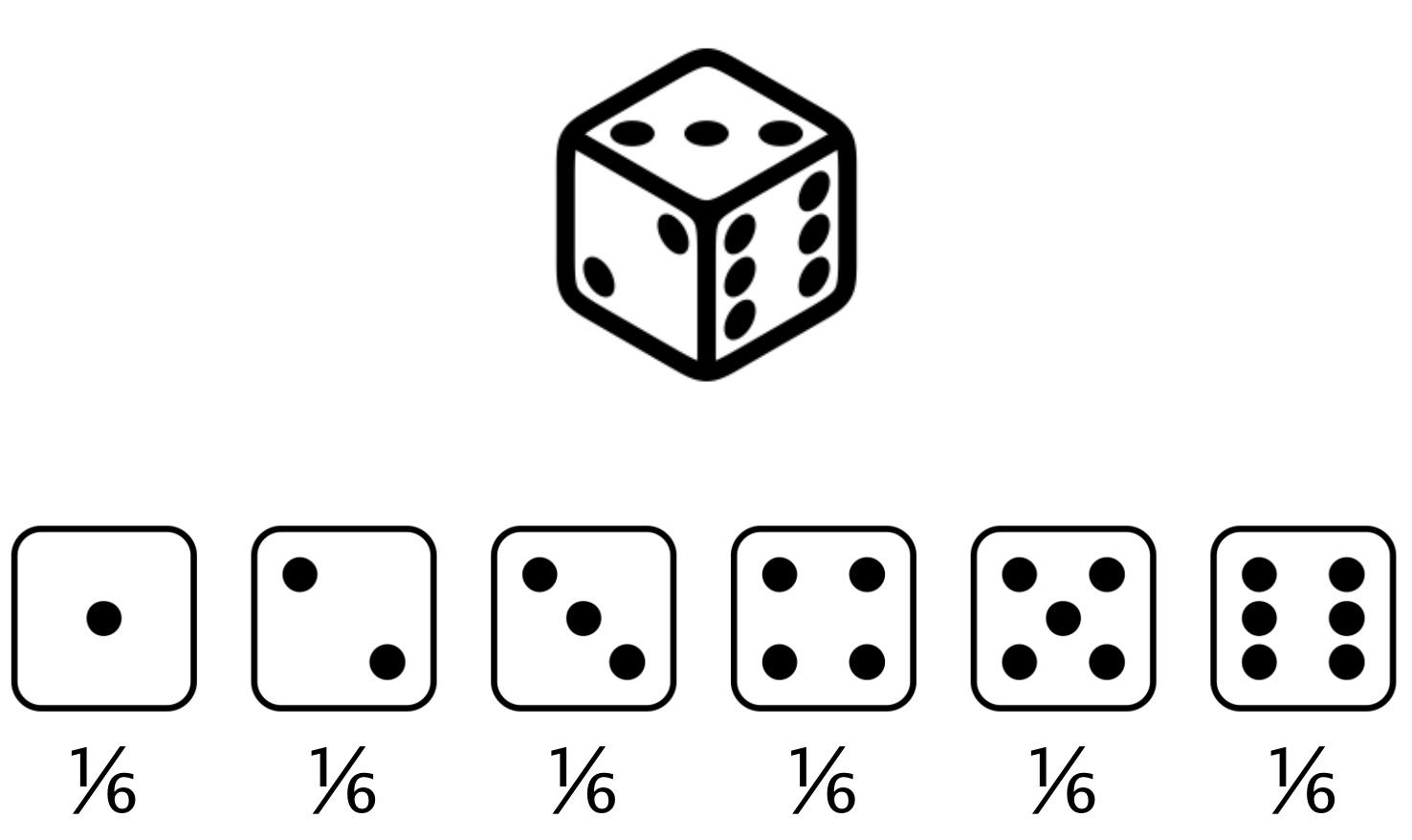
Probability distribution
Describes the probability of each possible outcome in a scenario
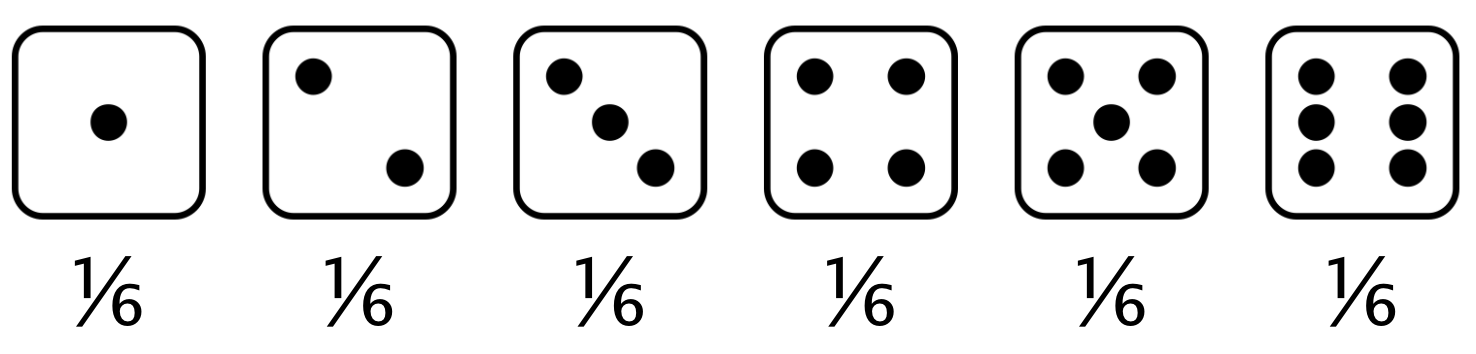
Expected value: mean of a probability distribution
Expected value of a fair die roll = $(1 \times \frac{1}{6}) + (2 \times \frac{1}{6}) +(3 \times \frac{1}{6}) +(4 \times \frac{1}{6}) +(5 \times \frac{1}{6}) +(6 \times \frac{1}{6}) = 3.5$
Visualizing a probability distribution
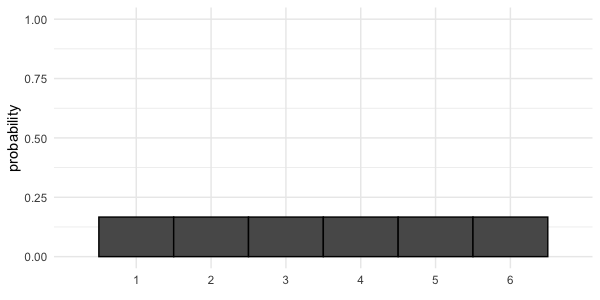
Probability = area
$$P(\text{die roll}) \le 2 = ~?$$
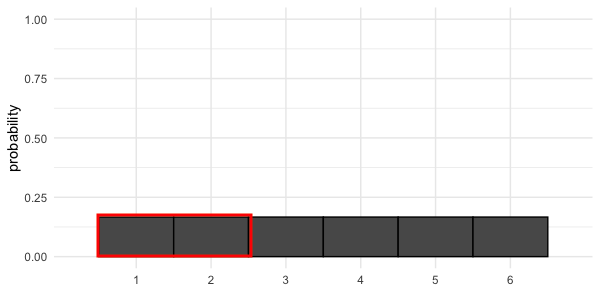
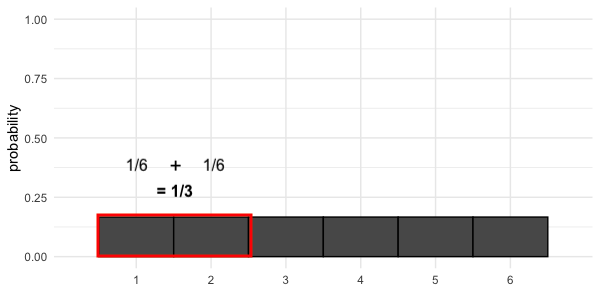
Probability = area
$$P(\text{die roll}) \le 2 = 1/3$$
Uneven die
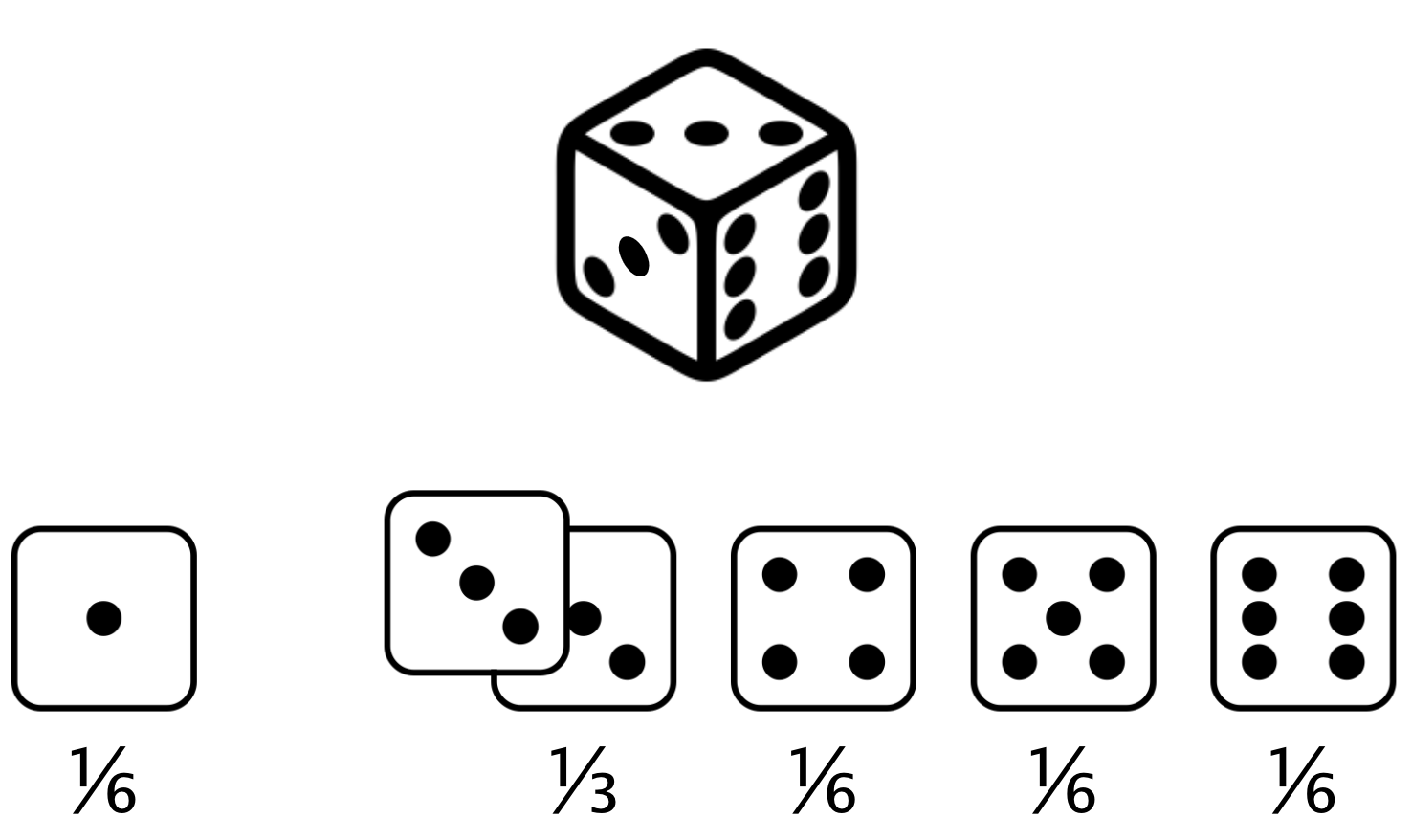
Expected value of uneven die roll = $(1 \times \frac{1}{6}) +(2 \times 0) +(3 \times \frac{1}{3}) +(4 \times \frac{1}{6}) +(5 \times \frac{1}{6}) +(6 \times \frac{1}{6}) = 3.67$
Visualizing uneven probabilities
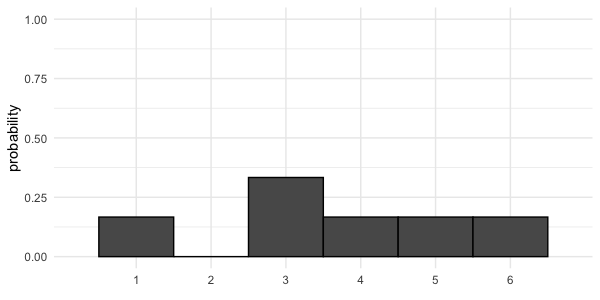
Adding areas
$$P(\text{uneven die roll}) \le 2 = ~?$$
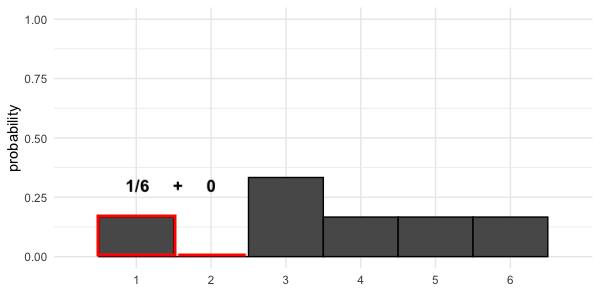
Adding areas
$$P(\text{uneven die roll}) \le 2 = 1/6$$

Discrete probability distributions
Describe probabilities for discrete outcomes
Fair die

Discrete uniform distribution
Uneven die
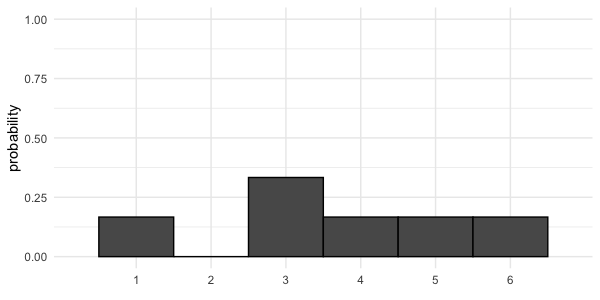
Sampling from discrete distributions
print(die)
number prob
0 1 0.166667
1 2 0.166667
2 3 0.166667
3 4 0.166667
4 5 0.166667
5 6 0.166667
np.mean(die['number'])
3.5
rolls_10 = die.sample(10, replace = True)
rolls_10
number prob
0 1 0.166667
0 1 0.166667
4 5 0.166667
1 2 0.166667
0 1 0.166667
0 1 0.166667
5 6 0.166667
5 6 0.166667
...
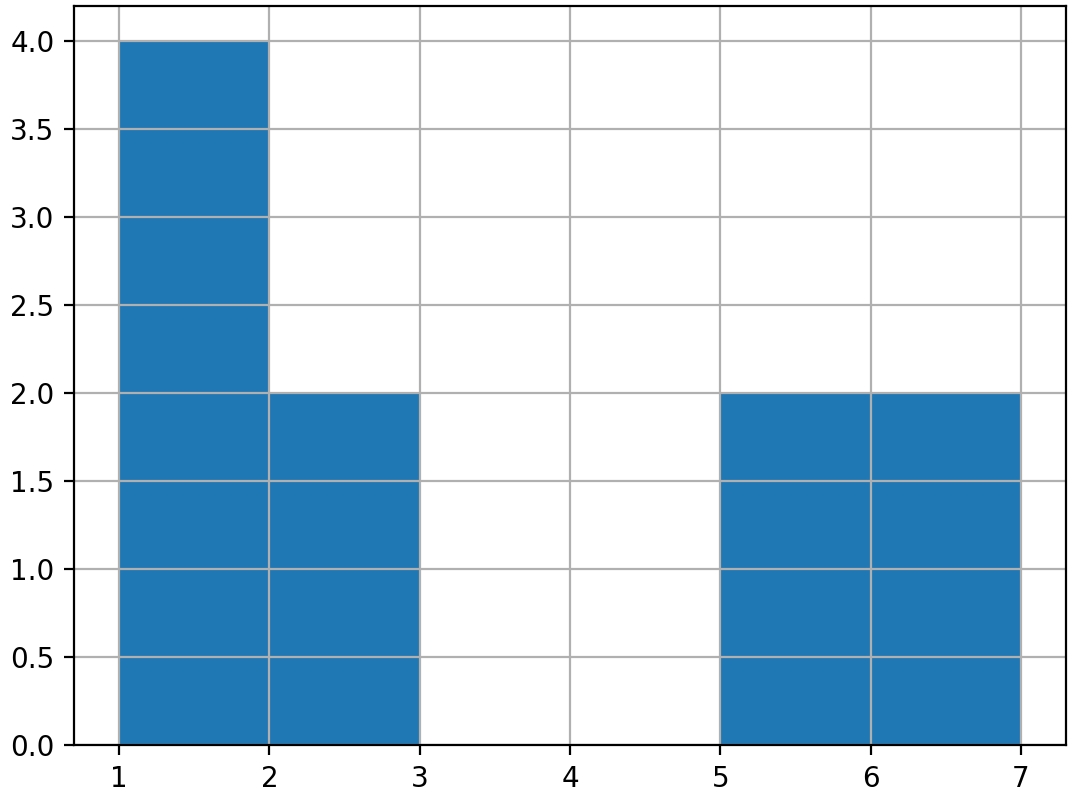
Visualizing a sample
rolls_10['number'].hist(bins=np.linspace(1,7,7))
plt.show()
Sample distribution vs. theoretical distribution
Sample of 10 rolls

np.mean(rolls_10['number']) = 3.0
Theoretical probability distribution
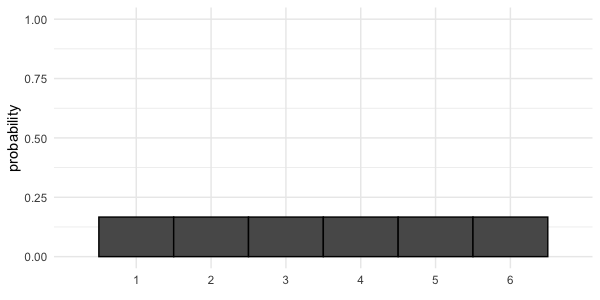
mean(die['number']) = 3.5
A bigger sample
Sample of 100 rolls
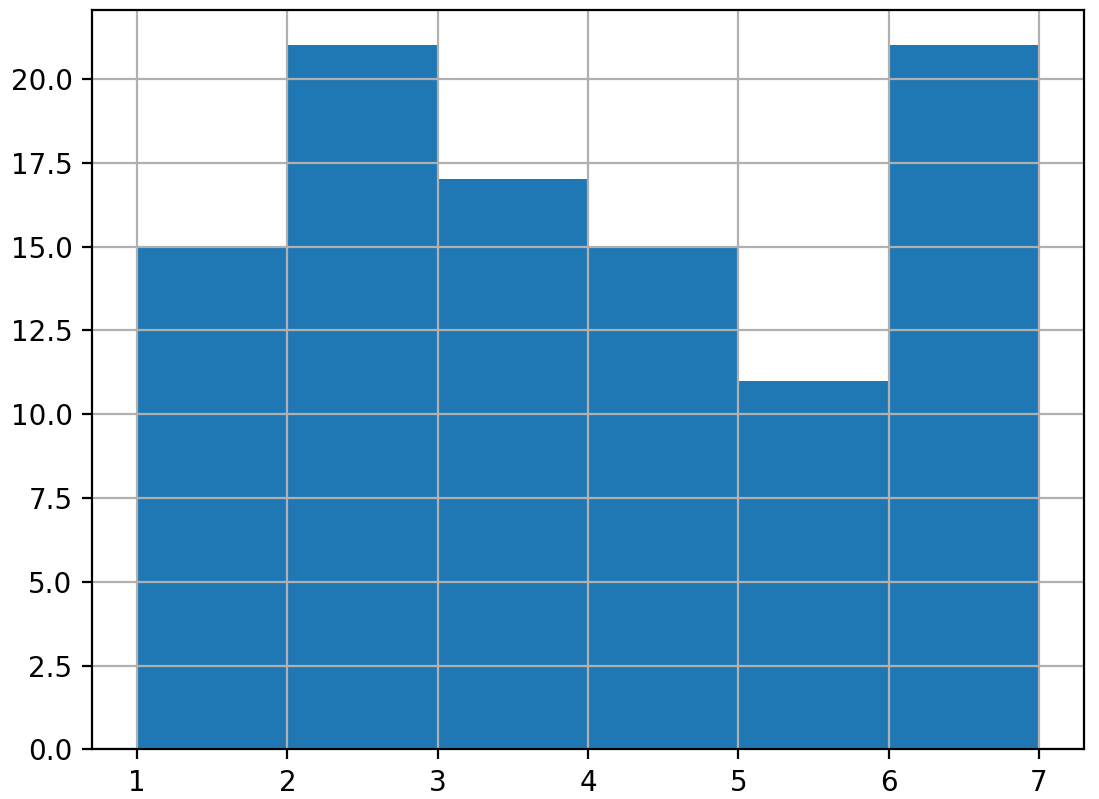
np.mean(rolls_100['number']) = 3.4
Theoretical probability distribution

mean(die['number']) = 3.5
An even bigger sample
Sample of 1000 rolls
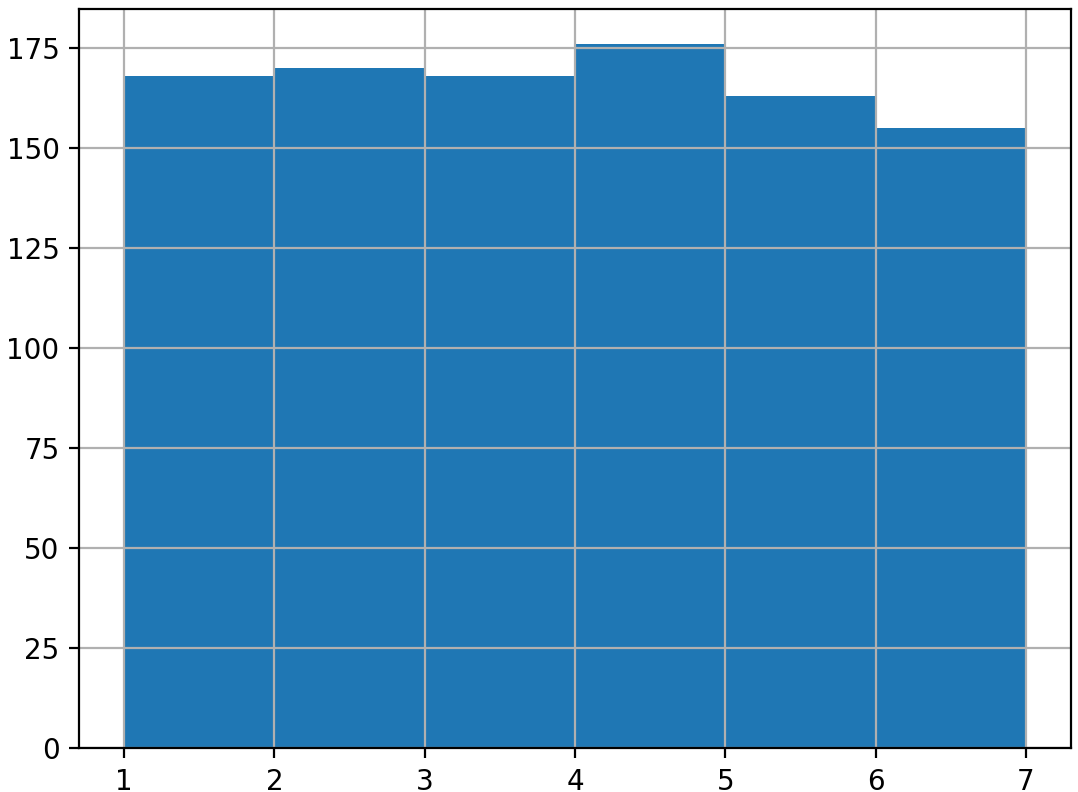
np.mean(rolls_1000['number']) = 3.48
Theoretical probability distribution

mean(die['number']) = 3.5
Law of large numbers
As the size of your sample increases, the sample mean will approach the expected value.
| Sample size | Mean |
|---|---|
| 10 | 3.00 |
| 100 | 3.40 |
| 1000 | 3.48 |
Let's practice!
Introduction to Statistics in Python

