Measures of spread
Introduction to Statistics

George Boorman
Curriculum Manager, DataCamp
What is spread?
Why is spread important?
- Spread measures the variety of our data
T-shirts typically cost $30
- Can cost between $10-200
- How likely is it one will cost $30?
If t-shirts were priced between $20-50
- Does this change the likelihood of finding one for $30?

1 Image credit: https://unsplash.com/@uyk
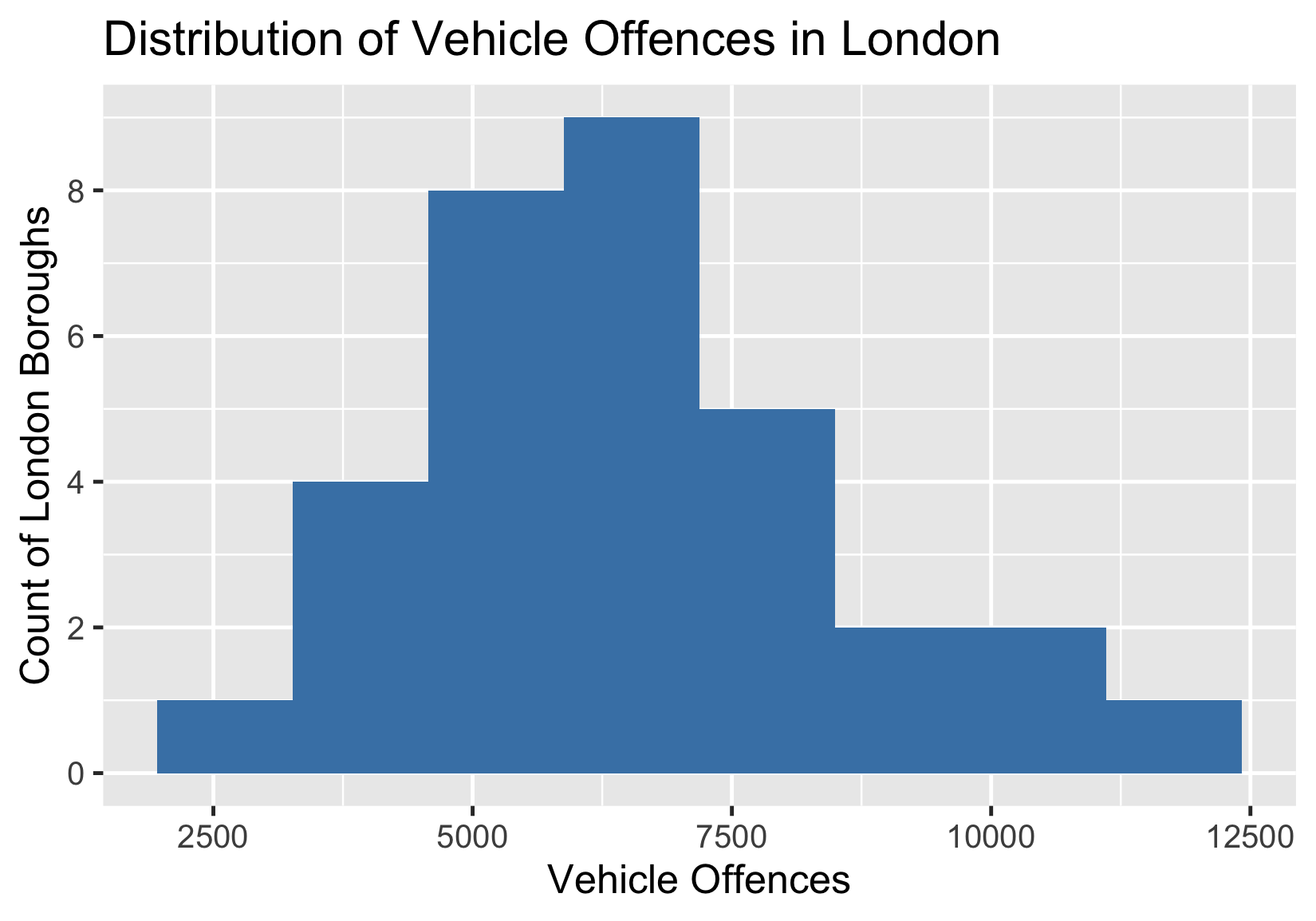
Range
${range} = maximum - minimum$
${range(Burglaries)} = 5,183 - 1,432$
${range(Burglaries)} = 3,751$
| Borough | Burglary |
|---|---|
| Tower Hamlets | 5,183 |
| Hackney | 5,079 |
| Barnet | 5,067 |
| ... | ... |
| Sutton | 1,815 |
| Bexley | 1,583 |
| Kingston upon Thames | 1,432 |
Variance
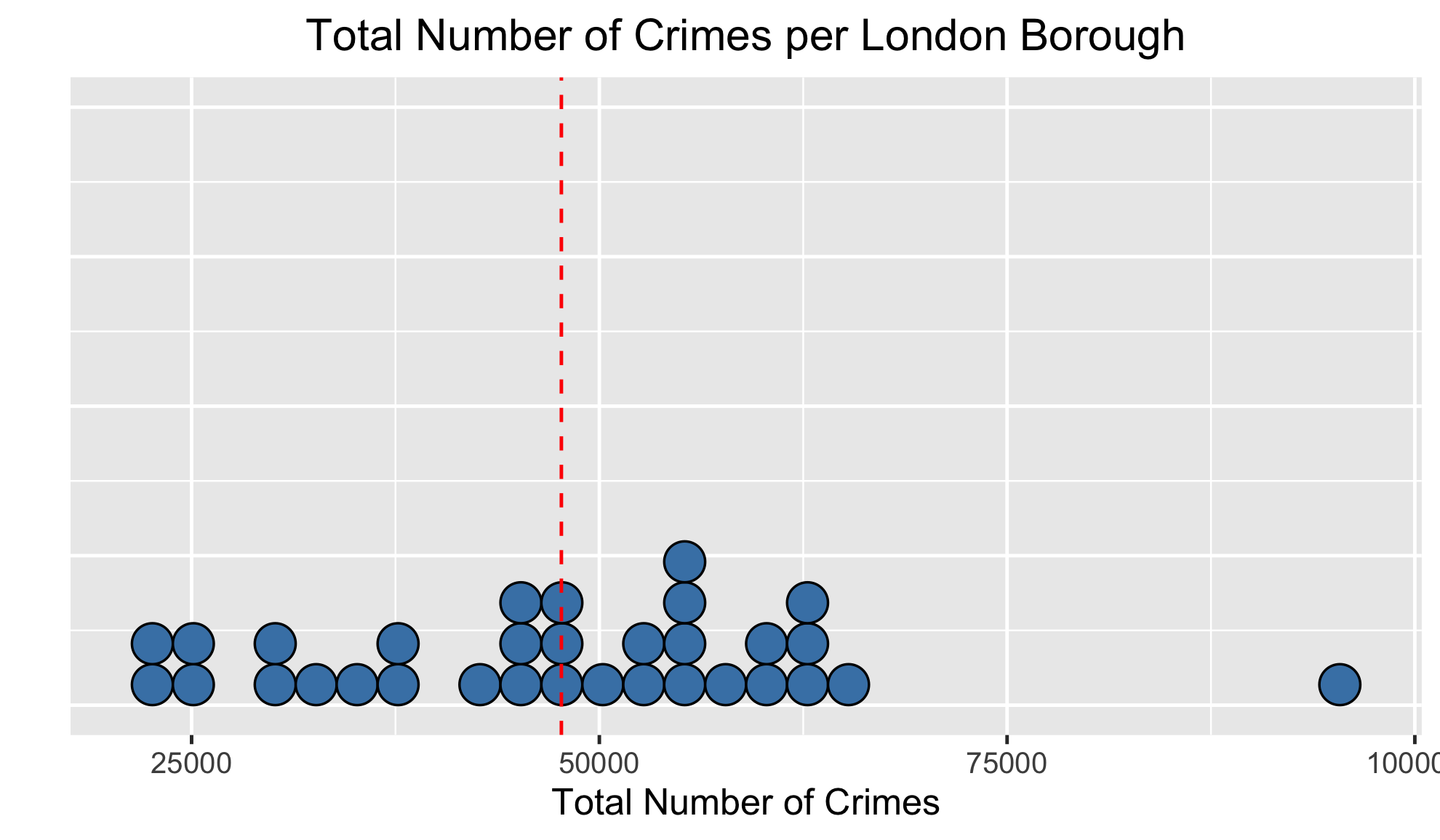
Variance
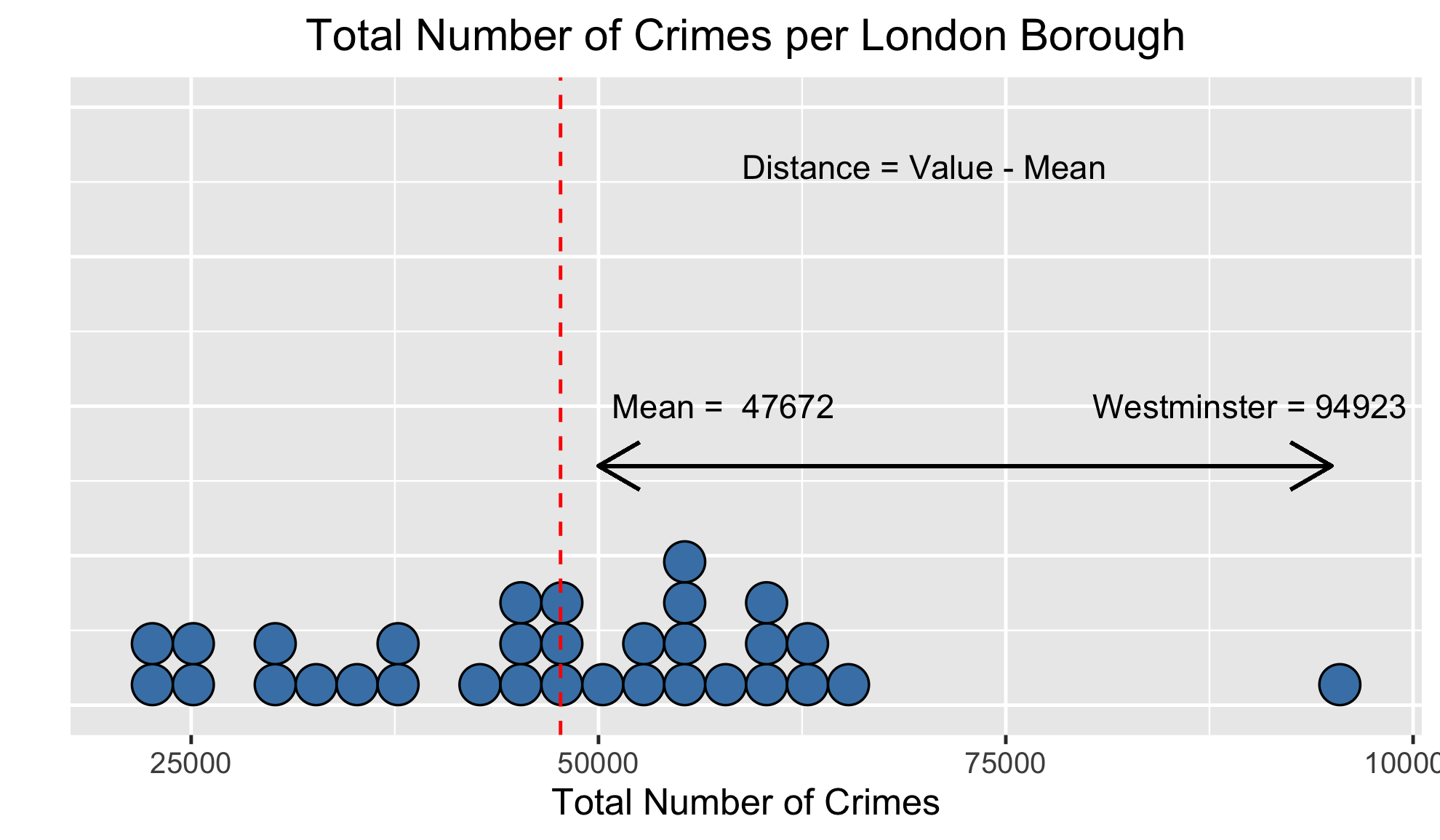
Variance
| Borough | Total Crime | Mean | Distance |
|---|---|---|---|
| Barking and Dagenham | 37,939 | 47,672 | -9,733 |
| Barnet | 52,421 | 47,672 | 4,749 |
| Bexley | 29,285 | 47,672 | -18,387 |
| Brent | 55,465 | 47,672 | 7,793 |
| Bromley | 42,982 | 47,672 | -4,690 |
| Camden | 54,806 | 47,672 | 7,134 |
| ... | ... | ... | ... |
| Total | 1,525,492 | 1,525,492 | 0 |
Variance
| Borough | Total Crime | Mean | Distance | Squared Distance |
|---|---|---|---|---|
| Barking and Dagenham | 37,939 | 47,672 | -9,733 | 94,731,289 |
| Barnet | 52,421 | 47,672 | 4,749 | 22,553,001 |
| Bexley | 29,285 | 47,672 | -18,387 | 338,081,769 |
| Brent | 55,465 | 47,672 | 7,793 | 60,730,849 |
| Bromley | 42,982 | 47,672 | -4,690 | 21,996,100 |
| Camden | 54,806 | 47,672 | 7,134 | 50,893,956 |
| ... | ... | ... | ... | ... |
| Total | 1,525,492 | 1,525,492 | 0 | 7,509,750,824 |
Variance
$${variance(total \ crime)} = \frac{7,509,750,824}{32}$$
$${variance(total \ crime)} = \ 234,679,713$$
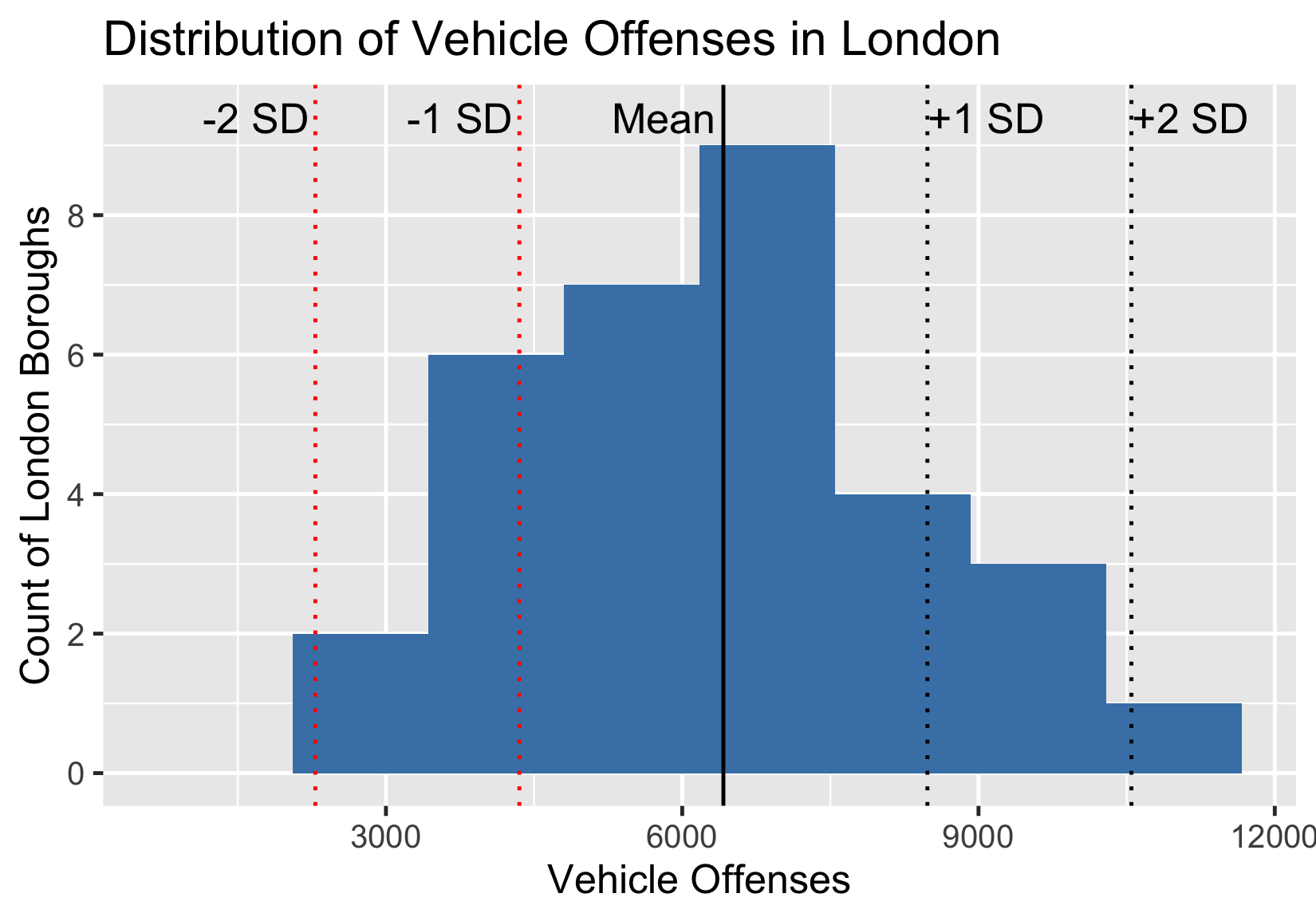
Standard deviation
${standard \ deviation(total \ crime)} = {\sqrt( variance(total \ crime))}$
${standard \ deviation(total \ crime)} = {\sqrt(234,679,713)}$
${standard \ deviation(total \ crime)} = 15,319.26$
- Standard deviation close to zero = data clustered around the mean
Standard deviation in a histogram
Quartiles
- Quartiles:
- splitting the data into four equal parts
| Crime | 0% | 25% | 50% | 75% | 100% |
|---|---|---|---|---|---|
| Burglary | 1,432.00 | 2,681.75 | 3,416.50 | 4,392.00 | 5,183.00 |
| Robbery | 363.00 | 895.75 | 1,354.50 | 1,976.50 | 4,156.00 |
| Theft | 4,090.00 | 7,739.75 | 9,624.00 | 12,059.00 | 40,278.00 |
| Vehicle Offenses | 2,143.00 | 4,838.25 | 6,424.50 | 7,520.75 | 11,292.00 |
Quartiles
- Quartiles:
- splitting the data into four equal parts
| Crime | 0% | 25% | 50% | 75% | 100% |
|---|---|---|---|---|---|
| Burglary | 1,432.00 | 2,681.75 | 3,416.50 | 4,392.00 | 5,183.00 |
| Robbery | 363.00 | 895.75 | 1,354.50 | 1,976.50 | 4,156.00 |
| Theft | 4,090.00 | 7,739.75 | 9,624.00 | 12,059.00 | 40,278.00 |
| Vehicle Offenses | 2,143.00 | 4,838.25 | 6,424.50 | 7,520.75 | 11,292.00 |
- Second quartile (50%) = median
Box plots

Interquartile range (IQR)
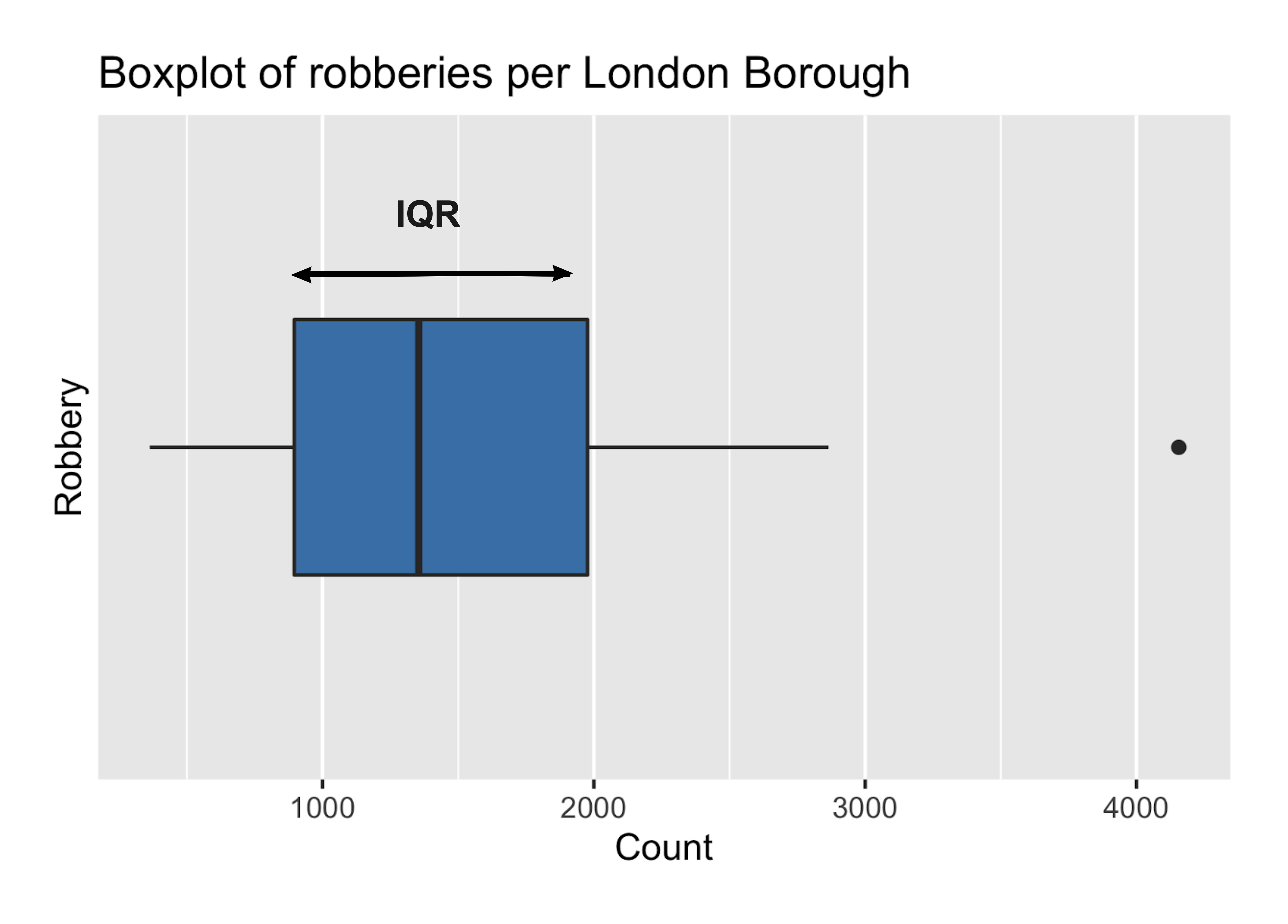
- IQR is less affected by extreme values
IQR = 3rd Quartile - 1st Quartile
IQR = 1976.50 - 895.75
IQR = 1080.75
Let's practice!
Introduction to Statistics

